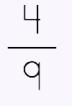|
||||||||||||||||||||||
 |
 |
 |
||||||
 |
||||||||
Ooooh…exponents! Back in elementary school, exponents were basically those little bitty numbers that were found at the tops of some numbers when you were working with area. In middle school, we begin using exponents in a whole new way and with other stuff besides area! In fact, you HAVE to have a good understanding of exponents because you are going to be seeing LOTS of 'em in Algebra so, get used to them (or, get over it!). Besides, they're not all THAT hard, after all… |
||
Let's say we're multiplying something like: |
||||
This is NOT the same as saying or writing: |
||||
Why? Well, 6 • 9 means "six groups of 9" and what we have is NOT six groups of nine, right? What we have is nine sixes and they are each multiplied by each other! This is where we can use the exponent. |
||
|
|||||||||||||||||||||||||
 |
||
Numbers with exponents are their own terms, so you need to think of them like they are their very own mini expressions in parentheses. In fact, they are very high on the 'to do' list in the Order of Operations; they are second only to grouping in the whole scheme of things (think GEMA). If you stop and think about it, it makes perfect sense: you ARE, after all, performing an operation! (all that multiplying? You betcha!) |
||
Whoa! A FRACTION as an exponent? What?! Yep, there IS such as thing as a fraction in the exponent! You wouldn't believe it but, it is true… Okay, let's back up a bit, shall we? First, let's go back to the regular ol' exponent and we'll backtrack a bit so it makes more sense. IF you have an exponent that is a 2, we call that 'squared.' Why? Because it makes a SQUARE, silly! |
||
When you take a number and you square it (the exponent is a 2), then we read the number as 'squared.' For example, |
||
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Now, if you want to go BACKWARDS when it comes to exponents, you have to be radical! Woo-hoo! Yep, that's right! RADICAL! No, seriously… |
||
The inverse of exponents is radical…or, to be more mathematical…taking the 'square root.' Awww…sorry to burst your bubble. The symbol is the radical sign (cool, right?) but, most people call it the square root sign. |
||
Well, the radical sign is the INVERSE operation of squaring! That's right! Let's say you square the number 2. What do you get? 4. So, the square root of 4 is 2 (i.e., √4 = 2)! What about if you take 3 and you square it. You get…9, right? If you take the square root of 9 (i.e., √9 =…), you get…3! Isn't that easy-peasy?Absolutely! |
||||
 |
||||
The amazing thing is that this can be re-written as a FRACTION! You got it…a FRACTION! |
||
Instead of writing √4 you can re-write this as : |
||||
If you have a fraction and you are raising it to a power, what do you THINK you might have to do? I bet you can reason this one out… |
||
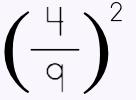 |
||
 |
||||
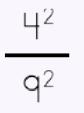
Did you come up with,
|
||||
|
||||||||||||||||||||||||||||||||||
You are SOOOOOO right! Yay! But, then, guess what…there's another way, of course… |
||||||||||||||||||||||||||||||||||
So, here is what you do:
If you did, then you would be correct! Woooo-hooo! So, what did you end up with when you FINALLY evaluated this one? hmm? |
||||
 |
||||
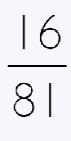 |
||||
Way to go! |
||||
©2011–2017 Sherry Skipper Spurgeon. All rights reserved. |
||